Last updated on April 25th, 2018 at 03:41 pm
How to Calculate Your Roof Area
Knowing how to calculate your roof area can be a beneficial first step in estimating the cost of a new metal roof. In roofing we call the calculation of roofing materials needed a “take-off”.
A good roofing contractor will take the time make sure that the take-off is accurate. Proper measurements and attention to roof details ensure that material shortages are avoided (shortages slow down installation) and prevent overages (overages add on unnecessary cost for the homeowner).
Why Should I Measure My Own Roof?
If you plan on re-roofing your own home then you will need to calculate materials so you won’t waste your own time and money. If you’re like most people, you’ll need to hire a professional.
Knowing your numbers will help you figure out what roofing materials are within your budget and having your square footage handy is a great way to protect yourself from inflated quotes.
Tips for Getting Started
Tip 1: Measuring to the nearest inch along eaves and across gables can be done from the ground – just don’t forget the overhang.
Tip 2: If you know the dimensions of your previous asphalt roofing job, you can use this info to cheat a little. Typical 3 Tab asphalt shingles have tabs that are 1 foot wide with a vertical exposure of 5 inches. Make sure to physically verify these dimensions by measuring 4 or 5 courses. Counting the shingles can give you eave to ridge dimensions from the ground.
Tip 3: 100 Square Feet = 1 Square in Roofing Terms
What You’ll Need
- 100’ tape measurer with grout hook (in Canada building products are still sold in square feet)
- Graph paper with ¼” squares on a clipboard
- Pencil and eraser
- Pitch finder tool (sold in most hardware stores) or pitch calculator app for your smart phone
Step 1: Sketch Roof Outline
Sketch the roof outline using ground measurements as shown in Figure 1 below. Knowing the longest dimension first will help you to scale your drawing appropriately.
Step 2: Fill in Roof Details
Measure and sketch out dormers, hips, valleys, diagonal distances and protrusions or overhangs. Chimneys, vents, skylights and pipe sizes will need to be measured to calculate trims and flashings needed for the job.
Pro Tip: You may need binoculars to count units of existing roof materials in unreachable areas. If different pitches exist on adjacent sides of a valley or hip, it’s length can be determined by treating the hip or valley as the diagonal (hypotenuse) of a right triangle on the roof pane next to it.
Step 3: Determine Roof Pitch
Pitch refers to the angle of the roof, or how steep it is. Measured by inches of rise (vertical measure) over 12 inches of run (horizontal measure) In the gable diagram in figure 2. (below) there are 4 units of rise and 6 units of run. This can be converted to units of rise over 12 by understanding that cross products in step 2 must be equal. There Therefore 4 x 12 must equal 6 x X. Step 3 shows that X must equal 48 divided by 6. Therefore X equals 8 and the pitch is 8/12.
A Note About Pythagorean Theorem (Yes, You Need It in Real Life)
If you know the measurement of two sides of a right triangle, but not the third side, then the Pythagorean Theorem from 8th Grade geometry is your best friend. In Figure 2 above, rise squared (multiplied by itself) plus run squared equals the diagonal squared. In the diagram, a2 + b2 = c2. If the rise is 42 + 62 = c2, then 16 + 36 = c2 – So c = the square root of 52. Pythagorean Theorem tells us that the gable (diagonal) length is 7.21’ or about 7’ 3”.
Step 4: Calculate The Simple Roof Areas
On a simple hip or gable roof, multiplying the eave to ridge length by eave length will give the area to be multiplied by pitch factor. Ridge vent openings are best finished with coil rather than partial shingle. Using flat coil stock allows the ridge caps to lay flat and can also be formed with an extra water stop at the ridge opening.
Area for the roof in Figure 3 is 1784 ft2 The blue dash line divides the roof into 2 rectangles. There is a small area calculated as 8/12 that is actually 4/12. On a small area such as this, it is better to err on the side of the higher pitch since it should not significantly affect the total.
Pro Tip: Pythagorean Theorem can be used to calculate the length of the valleys. A general rule of thumb is to add at least 12” lineal inches of valley material to be safe.
Step 5: Calculate The Complex Roof Areas
Most roofs in Canada would be considered on the complex side. Multiple adjacent pitches, dormers, and turrets are common. Keep in mind that an experienced metal roofing contractor will need to factor in extended labour time for areas of your roof that will require precision metalworking skills.
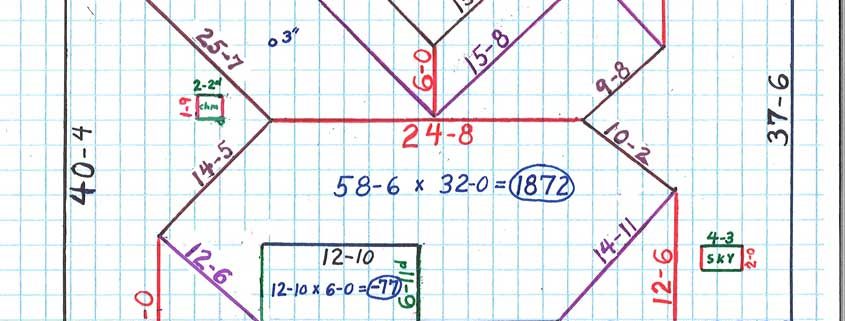
Start by finding the “footprint” area and multiply by the correct slope factor determined by pitch (see Pitch Factor Chart bottom of page). In Figure 4 below, the dashed blue lines give a simplified view of the “footprint”. Multiply L x W to calculate the area for each rectangular section. Note the area of 77ft2 that needs to be subtracted for the recessed area.
Once the “footprint” including overhang areas have been determined for each area, find the appropriate slope or pitch factor in the chart below. For example, a roof area with an 8/12 pitch has a slope factor of 1.202 so 2053ft2 x 1.202 = 2468ft2 total roof area.
A Note about complex roofs: Complex roofs need more accessories and trims than“easy” roofs – resulting in a slightly higher total cost/square foot.
Step 6: Measuring or Calculating Gable and Hip Lengths
The slope factor is also used to determine gable lengths when the run length is known. For example, in Figure 4 the bottom left gable measures 16’ across from eave to eave and therefore has a run of 8’. When this is multiplied by 1.202 the gable distance is 9’8” (9.62’ rounded up to nearest inch). Note that this run can also be multiplied by the hip/valley factor of 1.563 to get a valley length of 12’6”.
Record all your calculations on your drawing as seen in Figure 5 below.
Pro Tip: A Note About Waste Factor
Once you have figured out your roof area, you will need to also calculate how much room you need to allow for waste. For metal roofing products on a medium complex roof (common in Canada) a waste factor of 15% is advisable. In other words for the 2468 ft2 roof in our example you should multiply by 1.15 giving you 2838.2 ft2 roofing material needed.
Important Note: Most metal roofing products are packaged and sold by the square (100 square feet) so for our example roof the number of squares or boxes to purchase is 29.
| Run = Eave to Center Pt. | Pitch X/12 | Degrees | Slope Factor | Gable or Diagonal | Hip/Valley Factor | Hip or Valley |
|---|---|---|---|---|---|---|
| 3/12 | 14.0 | 1.031 | 1.436 | |||
| 4/12 | 18.4 | 1.054 | 1.453 | |||
| 5/12 | 22.6 | 1.083 | 1.474 | |||
| 10′ | 5/12 Example | 1.083 | 10′ 10″ | 1.474 | 14′ | |
| 6/12 | 26.6 | 1.118 | 1.500 | |||
| 7/12 Non-Walkable | 30.3 | 1.158 | 1.530 | |||
| 8/12 Non-Walkable | 33.7 | 1.202 | 1.563 | |||
| 10′ | 8/12 Example | 1.202 | 12′ | 1.563 | 15′ 8″ | |
| 9/12 Non-Walkable | 36.9 | 1.250 | 1.603 | |||
| 10/12 Non-Walkable | 39.8 | 1.302 | 1.641 | |||
| 11/12 Non-Walkable | 42.5 | 1.357 | 1.685 | |||
| 12/12 Non-Walkable | 45.0 | 1.414 | 1.732 | |||
| 10′ | 12/12 Example | 1.414 | 14′ 2″ | 1.732 | 17′ 4″ | |
| 13/12 Non-Walkable | 47.3 | 1.474 | 1.781 | |||
| 14/12 Non-Walkable | 49.4 | 1.537 | 1.833 | |||
| 15/12 Non-Walkable | 51.3 | 1.600 | 1.887 | |||
| 16/12 Non-Walkable | 53.1 | 1.667 | 1.944 |
PDF Download of Slope Factor Table
test








Leave a Reply
Want to join the discussion?Feel free to contribute!